Resistors are very important component in electronic circuit designing. Unlike inductors and capacitor Resistors are only component which possesses real power. A circuit only consisting of reactive element necessarily posses no power. Although those circuits are impossible to build. But this is also true that without capacitor and inductor also important electronic circuit elements. They are used in making filters and many more applications are there of these passive elements.
Summarized we can say that all the three passive elements are equally useful as the active elements are.
All values of passive elements are impossible to manufacture so the networks of passive elements are used for fulfillment of circuit requirement. There are various kind of passive networks some of them are as follows:
1. Serial Combination
2. Parallel Combination
3. Δ Combination
4. Y Combination
5. π Combination
And many more circuit combinations are there, for the fulfillment of circuit requirement.
Most commonly used combinations are Serial and Parallel combinations. Where as Δ and Y connections are at there place.
Δ and Y connections: When the circuit elements are arranged in such a manner, as shown in figure below, are called as Δ and Y combinations of passive elements. Y connection often called as Star connection.
The interconversion between these networks is also necessary.
For the interconversion we assume that the voltage at the corners of both connection are equal and total resistance is also equal.
Because both the circuit are identical so current flowing between terminals V1 and V2 is equal, and it is equal only when effective resistance between them is equal. So using this we can write that
Impedance b/w V1 and V2 in star = Impedance b/e V1 and V2 in Delta
Similarly from the cyclic symmetry, other branches impedance equation can also be written.
These are three impedance equation for three branches.
subtracting 2 from 1 we get
adding 3 and 4
This is first star impedance from the delta impedance. From the cyclic symmetry we can get other also:
That's all the delta to star conversion. Now the reverse conversion is also required.
After multiplying 5, 6, 7 in pairs and adding them we get
comparing equation 11 and 7 we get:
From equation 12 we get d1 and from the cyclic symmetry d2 and d3 can also be written as follows:
These are the delta impedance from the star impedance.
for more information click the below link.




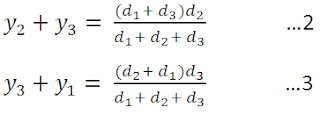









No comments:
Post a Comment
If the contents is insufficient or if there any error, please write here....
Suggestion are welcome: